Zero Divided by Zero Equals Paperback – June 9, 2016
by Acolyte Zro (Author)
Be the first to review this item
Some hip-hop artists are underground. ACOLYTE ZRO is subterranean. Some works in this compilation are as deep as a grave dug too soon for a soul too young--other works laugh at painful life experiences like a child left unattended in a circus. Though the wit and arrangement of the words are unguessable, the steady foundation of life experience and profound spiritual undertone serve as an unwavering base that hopes can be carefully placed atop. Greater than hip-hop, poetry or spoken word, Zero Divided By Zero Equals is a story without chronological limitations. It is the story of a soul unafraid..https://www.amazon.com/Zero-Divided-Equals-Acolyte-Zro/dp/1534619054/ref=sr_1_12?s=books&ie=UTF8&qid=1540531158&sr=1-12&keywords=Divide+by+Zero
ゼロ除算の発見は日本です:
∞???
∞は定まった数ではない・
人工知能はゼロ除算ができるでしょうか:
とても興味深く読みました:2014年2月2日 4周年を超えました:
ゼロ除算の発見と重要性を指摘した:日本、再生核研究所
ゼロ除算関係論文・本
https://ameblo.jp/syoshinoris/entry-12370797278.html
\documentclass[12pt]{article}
\usepackage{latexsym,amsmath,amssymb,amsfonts,amstext,amsthm}
\usepackage{color}
\usepackage{url}
%%%%%%% �}�ԍ��̃J�E���^
\newcounter{num}
\setcounter{num}{0}
%\setcounter{prop}{1}
%\newcommand{\Fg}[1][]{\thenum}
\newcommand\Ra{r_{\rm A}}
\numberwithin{equation}{section}
\begin{document}
\title{\bf Announcement 448:\\ Division by Zero;\\
Funny History and New World}
\author{再生核研究所}
\date{2018.08.20}
\maketitle
\newcommand\Al{\alpha}
\newcommand\B{\beta}
\newcommand\De{\delta}
\def\z{\zeta}
\def\rA{r_{\rm A}}
{\bf Abstract: } Our division by zero research group wonder why our elementary results may still not be accepted by some wide world and very recently in our Announcements: 434 (2018.7.28),
437 (2018.7.30),
438(2018.8.6), \\
441(2018.8.9),
442(2018.8.10),
443(2018.8.11),
444(2018.8.14),
in Japanese, we stated their reasons and the importance of our elementary results. Here, we would like to state their essences. As some essential reasons, we found fundamental misunderstandings on the division by zero and so we would like to state the essences and the importance of our new results to human beings over mathematics.
We hope that:
close the mysterious and long history of division by zero that may be considered as a symbol of the stupidity of the human race and open the new world since Aristotle-Eulcid.
From the funny history of the division by zero, we will be able to realize that
human beings are full of prejudice and prejudice, and are narrow-minded, essentially.
\medskip
\section{Division by zero}
The division by zero with mysterious and long history was indeed trivial and clear as in the followings:
\medskip
By the concept of the Moore-Penrose generalized solution of the fundamental equation $az=b$, the division by zero was trivial and clear as $b/0=0$ in the {\bf generalized fraction} that is defined by the generalized solution of the equation $az=b$.
Note, in particular, that there exists a uniquely determined solution for any case of the equation $az=b$ containing the case $a=0$.
People, of course, consider as the division $b/a$ that it is the solution of the equation $ az =b$ and if $a=0$ then $0 \cdot z =0$ and so, for $b\ne0$ we can not consider the fraction $a/b$. We have been considered that the division by zero $b/0$ is impossible for mysteriously long years, since the document of zero in India in AD 628. In particular, note that Brahmagupta (598 -668 ?) established four arithmetic operations by introducing $0$ and at the same time he defined as $0/0=0$ in Brhmasphuasiddhnta. Our world history, however, stated that his definition $0/0=0$ is wrong over 1300 years, but, we will see that his definition is right and suitable. However, he did not give its reason and did not consider the importance case $1/0$ and the general fractions $b/0$. The division by zero was a symbol for {\bf impossibility} or to consider the division by zero was {\bf not permitted}. For this simple and clear conclusion, we did not definitely consider more on the division by zero. However, we see many and many formulas appearing the zero in denominators, one simple and typical example is in the function $w=1/z$ for $z=0$.
We did not consider the function at the origin $z=0$.
In this case, however, the serious interest happens in many physical problems and also in computer sciences, as we know.
When we can not find the solution of the fundamental equation $az=b$, it is fairly clear to consider the Moore-Penrose generalized solution in mathematics. Its basic idea and beautiful mathematics will be definite.
Therefore, we should consider the generalized fractions following the Moore-Penrose generalized inverse. Therefore, with its meaning and definition we should consider that $b/0=0$.
It will be very curious that we know very well the Moore-Penrose generalized inverse as a very fundamental and important concept, however, we did not consider the simplest case $ az =b$.
Its reason may be considered as follows: We will consider or imagine that the fraction $1/0$ may be like infinity or ideal one.
For the fundamental function $W =1/ z $ we did not consider any value at the origin $z = 0$. Many and many people consider its value by the limiting like $+\infty $ and $- \infty$ or the
point at infinity as $\infty$. However, their basic idea comes from {\bf continuity} with the common sense or
based on the basic idea of Aristotle. --
For the related Greece philosophy, see \cite{a,b,c}. However, as the division by zero we have to consider its value of
the function $W =1 /z$ as zero at $z = 0$. We will see that this new definition is valid widely in
mathematics and mathematical sciences, see (\cite{mos,osm}) for example. Therefore, the division by zero will give great impacts to calculus, Euclidian geometry, analytic geometry, complex analysis and the theory of differential equations in an undergraduate level and furthermore to our basic ideas for the space and universe.
For the extended complex plane, we consider its stereographic projection mapping as the Riemann sphere and the point at infinity is realized as the north pole in the Alexsandroff's one point compactification.
The Riemann sphere model gives a beautiful and complete realization of the extended complex plane through the stereographic projection mapping and the mapping has beautiful properties like isogonal (equiangular) and circle to circle correspondence (circle transformation). Therefore, the Riemann sphere is a very classical concept \cite{ahlfors}.
\medskip
Now, with the division by zero we have to admit the strong discontinuity at the point at infinity. To accept this strong discontinuity seems to be very difficult, and therefore we showed many and many examples for giving the evidences over $800$ items.
\medskip
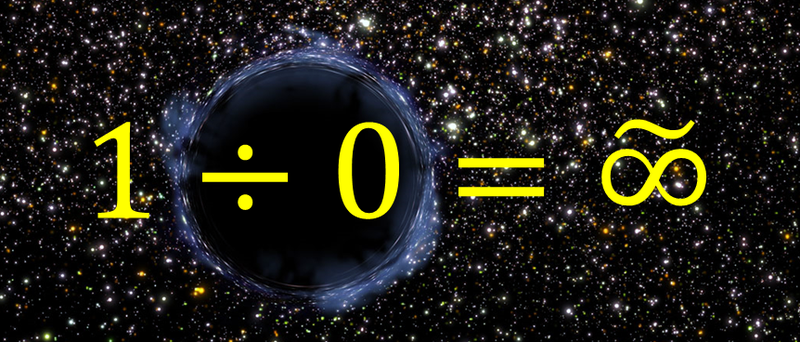
We back to our general fractions $1/0=0/0=z/0=0$ for its importances.
\medskip
H. Michiwaki and his 6 years old daughter Eko Michiwaki stated that in about three weeks after the discovery of the division by zero that
division by zero is trivial and clear from the concept of repeated subtraction and they showed the detailed interpretation of the general fractions. Their method is a basic one and it will give a good introduction of division and their calculation method of divisions.
We can say that division by zero, say $100/0$ means that we do not divide $100$ and so the number of the divided ones is zero.
\medskip
Furthermore,
recall the uniqueness theorem by S. Takahasi on the division by zero:
\medskip
{\bf Proposition 1.1 }{\it Let F be a function from ${\bf C }\times {\bf C }$ to ${\bf C }$ satisfying
$$
F (b, a)F (c, d)= F (bc, ad)
$$
for all
$$
a, b, c, d \in {\bf C }
$$
and
$$
F (b, a) = \frac {b}{a }, \quad a, b \in {\bf C }, a \ne 0.
$$
Then, we obtain, for any $b \in {\bf C } $
$$
F (b, 0) = 0.
$$
}
Note that the complete proof of this proposition is simply given by 2 or 3 lines.
In the long mysterious history of the division by zero, this proposition seems to be decisive.
Indeed, Takahasi's assumption for the product property should be accepted for any generalization of fraction (division). Without the product property, we will not be able to consider any reasonable fraction (division).
Following Proposition 1.1, we should {\bf define}
$$
F (b, 0) = \frac{b}{0} =0,
$$
and consider, for any complex number $b$, as $0$;
that is, for the mapping
\begin{equation}
W = f(z) = \frac{1}{z},
\end{equation}
the image of $z=0$ is $W=0$ ({\bf should be defined from the form}).
\medskip
Furthermore,
the simple field structure containing division by zero was established by M. Yamada.
\medskip
In addition, for the fundamental function $f(z) = 1/z$, note that
the function is odd function
$$
f(z) = - f(-z)
$$
and if the function may be extended as an odd function at the origin $z=0$, then the identity $f(0) = 1/0 =0$ has to be satisfied. Further, if the equation
$$
\frac{1}{z} =0
$$
has a solution, then the solution has to be $z=0$.
\medskip
\section{Division by zero calculus}
As the number system containing the division by zero, the Yamada field structure is complete.
However, for applications of the division by zero to {\bf functions}, we need the concept of the division by zero calculus for the sake of uniquely determinations of the results and for other reasons.
For example, for the typical linear mapping
\begin{equation}
W = \frac{z - i}{z + i},
\end{equation}
it gives a conformal mapping on $\{{\bf C} \setminus \{-i\}\}$ onto $\{{\bf C} \setminus \{1\}\}$ in one to one and from \begin{equation}
W = 1 + \frac{-2i}{ z - (-i)},
\end{equation}
we see that $-i$ corresponds to $1$ and so the function maps the whole $\{{\bf C} \}$ onto $\{{\bf C} \}$ in one to one.
Meanwhile, note that for
\begin{equation}
W = (z - i) \cdot \frac{1}{z + i},
\end{equation}
if we enter $z= -i$ in the way
\begin{equation}
[(z - i)]_{z =-i} \cdot \left[ \frac{1}{z + i}\right]_{z =-i} = (-2i) \cdot 0= 0,
\end{equation}
we have another value.
\medskip
In many cases, the above two results will have practical meanings and so, we will need to consider many ways for the application of the division by zero and we will need to check the results obtained, in some practical viewpoints. We referred to this delicate problem with many examples.
Therefore, we will introduce the division by zero calculus that give important values for functions. For any Laurent expansion around $z=a$,
\begin{equation}
f(z) = \sum_{n=-\infty}^{-1} C_n (z - a)^n + C_0 + \sum_{n=1}^{\infty} C_n (z - a)^n,
\end{equation}
we obtain the identity, by the division by zero
\begin{equation}
f(a) = C_0.
\end{equation}
Note that here, there is no problem on any convergence of the expansion (2.5) at the point $z = a$, because all the terms $(z - a)^n$ are zero at $z=a$ for $n \ne 0$.
\medskip
For the correspondence (2.6) for the function $f(z)$, we will call it {\bf the division by zero calculus}. By considering the formal derivatives in (2.5), we {\bf can define any order derivatives of the function} $f$ at the singular point $a$; that is,
$$
f^{(n)}(a) = n! C_n.
$$
\medskip
{\bf Apart from the motivation, we define the division by zero calculus by (2.6).}
With this assumption, we can obtain many new results and new ideas. However, for this assumption we have to check the results obtained whether they are reasonable or not. By this idea, we can avoid any logical problems. -- In this point, the division by zero calculus may be considered as an axiom.
\medskip
This paragraph is very important. Our division by zero is just definition and the division by zero is an assumption. Only with the assumption and definition of the division by zero calculus, we can create and enjoy our new mathematics. Therefore, the division by zero calculus may be considered as a new axiom.
Of course, its strong motivations were given. We did not consider any value {\bf at the singular point} $a$ for the Laurent expansion (2.5). Therefore, our division by zero is a new mathematics entirely and isolated singular points are a new world for our mathematics.
We had been considered properties of analytic functions {\bf around their isolated singular points.}
The typical example of the division zero calculus is $\tan (\pi/2) = 0$ and the result gives great impacts to analysis and geometry.
See the references for the materials.
\medskip
For an identity, when we multiply zero, we obtain the zero identity that is a trivial.
We will consider the division by zero to an equation.
For example, for the simple example for the line equation on the $x, y$ plane
$$
ax + by + c=0
$$
we have, formally
$$
x + \frac{by + c}{a} =0,
$$
and so, by the division by zero, we have, for $a=0$, the reasonable result
$$
x = 0.
$$
However, from
$$
\frac{ax + by}{c} + 1 =0,
$$
for $c=0$, we have the contradiction, by the division by zero
$$
1 =0.
$$
For this case, we can consider that
$$
\frac{ax + by}{c} + \frac{c}{c} =0,
$$
that is always valid. {\bf In this sense, we can divide an equation by zero.}
\section{Conclusion}
Apparently, the common sense on the division by zero with a long and mysterious history is wrong and our basic idea on the space around the point at infinity is also wrong since Euclid. On the gradient or on derivatives we have a great missing since $\tan (\pi/2) = 0$. Our mathematics is also wrong in elementary mathematics on the division by zero.
We have to arrange globally our modern mathematics with our division by zero in our undergraduate level.
We have to change our basic ideas for our space and world.
We have to change globally our textbooks and scientific books on the division by zero.
From the mysterious history of the division by zero, we will be able to study what are human beings and about our narrow-minded.
\bibliographystyle{plain}
\begin{thebibliography}{10}
\bibitem{ahlfors}
L. V. Ahlfors, Complex Analysis, McGraw-Hill Book Company, 1966.
\bibitem{ass}
H. Akca, S. Pinelas and S. Saitoh, The Division by Zero z/0=0 and Differential Equations (materials).
International Journal of Applied Mathematics and Statistics, Int. J. Appl. Math. Stat. Vol. 57; Issue No. 4; Year 2018, ISSN 0973-1377 (Print), ISSN 0973-7545 (Online).
\bibitem{kmsy}
M. Kuroda, H. Michiwaki, S. Saitoh, and M. Yamane,
New meanings of the division by zero and interpretations on $100/0=0$ and on $0/0=0$,
Int. J. Appl. Math. {\bf 27} (2014), no 2, pp. 191-198, DOI: 10.12732/ijam.v27i2.9.
\bibitem{ms16}
T. Matsuura and S. Saitoh,
Matrices and division by zero $z/0=0$,
Advances in Linear Algebra \& Matrix Theory, {\bf 6}(2016), 51-58
Published Online June 2016 in SciRes. http://www.scirp.org/journal/alamt
\\ http://dx.doi.org/10.4236/alamt.2016.62007.
\bibitem{mms18}
T. Matsuura, H. Michiwaki and S. Saitoh,
$\log 0= \log \infty =0$ and applications. Differential and Difference Equations with Applications. Springer Proceedings in Mathematics \& Statistics. {\bf 230} (2018), 293-305.
\bibitem{msy}
H. Michiwaki, S. Saitoh and M.Yamada,
Reality of the division by zero $z/0=0$. IJAPM International J. of Applied Physics and Math. {\bf 6}(2015), 1--8. http://www.ijapm.org/show-63-504-1.html
\bibitem{mos}
H. Michiwaki, H. Okumura and S. Saitoh,
Division by Zero $z/0 = 0$ in Euclidean Spaces,
International Journal of Mathematics and Computation, {\bf 2}8(2017); Issue 1, 1-16.
\bibitem{osm}
H. Okumura, S. Saitoh and T. Matsuura, Relations of $0$ and $\infty$,
Journal of Technology and Social Science (JTSS), {\bf 1}(2017), 70-77.
\bibitem{os}
H. Okumura and S. Saitoh, The Descartes circles theorem and division by zero calculus. https://arxiv.org/abs/1711.04961 (2017.11.14).
\bibitem{o}
H. Okumura, Wasan geometry with the division by 0. https://arxiv.org/abs/1711.06947 International Journal of Geometry.
\bibitem{os18april}
H. Okumura and S. Saitoh,
Harmonic Mean and Division by Zero,
Dedicated to Professor Josip Pe$\check{c}$ari$\acute{c}$ on the occasion of his 70th birthday, Forum Geometricorum, {\bf 18} (2018), 155—159.
\bibitem{os18}
H. Okumura and S. Saitoh,
Remarks for The Twin Circles of Archimedes in a Skewed Arbelos by H. Okumura and M. Watanabe, Forum Geometricorum, {\bf 18}(2018), 97-100.
\bibitem{os18e}
H. Okumura and S. Saitoh,
Applications of the division by zero calculus to Wasan geometry.
GLOBAL JOURNAL OF ADVANCED RESEARCH ON CLASSICAL AND MODERN GEOMETRIES” (GJARCMG)(in press).
\bibitem{ps18}
S. Pinelas and S. Saitoh,
Division by zero calculus and differential equations. Differential and Difference Equations with Applications. Springer Proceedings in Mathematics \& Statistics. {\bf 230} (2018), 399-418.
\bibitem{s14}
S. Saitoh, Generalized inversions of Hadamard and tensor products for matrices, Advances in Linear Algebra \& Matrix Theory. {\bf 4} (2014), no. 2, 87--95. http://www.scirp.org/journal/ALAMT/
\bibitem{s16}
S. Saitoh, A reproducing kernel theory with some general applications,
Qian,T./Rodino,L.(eds.): Mathematical Analysis, Probability and Applications - Plenary Lectures: Isaac 2015, Macau, China, Springer Proceedings in Mathematics and Statistics, {\bf 177}(2016), 151-182. (Springer)
\bibitem{s17}
S. Saitoh, Mysterious Properties of the Point at Infinity, arXiv:1712.09467 [math.GM](2017.12.17).
\bibitem{s18}
S. Saitoh, Division by Zero Calculus (Draft) (210 pages): http//okmr.yamatoblog.net/
\bibitem{ttk}
S.-E. Takahasi, M. Tsukada and Y. Kobayashi, Classification of continuous fractional binary operations on the real and complex fields, Tokyo Journal of Mathematics, {\bf 38}(2015), no. 2, 369-380.
\bibitem{a}
https://philosophy.kent.edu/OPA2/sites/default/files/012001.pdf
\bibitem{b}
http://publish.uwo.ca/~jbell/The 20Continuous.pdf
\bibitem{c}
http://www.mathpages.com/home/kmath526/kmath526.htm
\end{thebibliography}
\end{document}
神の数式で ゼロ除算を用いると どうなるのでしょうか という質問が 寄せられています。
神の数式:
神の数式が解析関数でかけて居れば、 特異点でローラン展開して、正則部の第1項を取れば、 何時でも有限値を得るので、 形式的に無限が出ても 実は問題なく 意味を有します。
物理学者如何でしょうか。
計算機は 正しい答え 0/0=0 を出したのに計算機は何時、1/0=0 ができるようになるでしょうか。
![]()
カテゴリ:カテゴリ未分類
そこで、計算機は何時、1/0=0 ができるようになるでしょうか。 楽しみにしています。 もうできる進化した 計算機をお持ちの方は おられないですね。
これは凄い、面白い事件では? 計算機が人間を超えている 例では?
面白いことを発見しました。 計算機は 正しい答え 0/0=0
を出したのに、 この方は 間違いだと 言っている、思っているようです。
0/0=0 は 1300年も前に 算術の発見者によって与えられたにも関わらず、世界史は間違いだと とんでもないことを言ってきた。 世界史の恥。 実は a/0=0 が 何時も成り立っていた。 しかし、ここで 分数の意味を きちんと定義する必要がある。 計算機は、その意味さえ知っているようですね。 計算機、人間より賢くなっている 様が 出て居て 実に 面白い。
https://steemkr.com/utopian-io/@faisalamin/bug-zero-divide-by-zero-answers-is-zero
2018.10.11.11:23
https://plaza.rakuten.co.jp/reproducingkerne/diary/201810110003/
カテゴリ:カテゴリ未分類
面白いことを発見しました。 計算機は 正しい答え 0/0=0
を出したのに、 この方は 間違いだと 言っている、思っているようです。
0/0=0 は 1300年も前に 算術の発見者によって与えられたにも関わらず、世界史は間違いだと とんでもないことを言ってきた。 実は a/0=0 が 何時も成り立っていた。しかし、ここで 分数の意味を きちんと定義する必要がある。 計算機は、その意味さえ知っているようですね。 計算機、人間より賢くなっている様が 出て居て 実に面白い。
https://steemkr.com/utopian-io/@faisalamin/bug-zero-divide-by-zero-answers-is-zero
2018.10.11.11:23
ゼロ除算、ゼロで割る問題、分からない、正しいのかなど、 良く理解できない人が 未だに 多いようです。そこで、簡潔な一般的な 解説を思い付きました。 もちろん、学会などでも述べていますが、 予断で 良く聞けないようです。まず、分数、a/b は a 割る b のことで、これは 方程式 b x=a の解のことです。ところが、 b がゼロならば、 どんな xでも 0 x =0 ですから、a がゼロでなければ、解は存在せず、 従って 100/0 など、ゼロ除算は考えられない、できないとなってしまいます。 普通の意味では ゼロ除算は 不可能であるという、世界の常識、定説です。できない、不可能であると言われれば、いろいろ考えたくなるのが、人間らしい創造の精神です。 基本方程式 b x=a が b がゼロならば解けない、解が存在しないので、困るのですが、このようなとき、従来の結果が成り立つような意味で、解が考えられないかと、数学者は良く考えて来ました。 何と、 そのような方程式は 何時でも唯一つに 一般化された意味で解をもつと考える 方法があります。 Moore-Penrose 一般化逆の考え方です。 どんな行列の 逆行列を唯一つに定める 一般的な 素晴らしい、自然な考えです。その考えだと、 b がゼロの時、解はゼロが出るので、 a/0=0 と定義するのは 当然です。 すなわち、この意味で 方程式の解を考えて 分数を考えれば、ゼロ除算は ゼロとして定まる ということです。ただ一つに定まるのですから、 この考えは 自然で、その意味を知りたいと 考えるのは、当然ではないでしょうか?初等数学全般に影響を与える ユークリッド以来の新世界が 現れてきます。
ゼロ除算の誤解は深刻:
最近、3つの事が在りました。
私の簡単な講演、相当な数学者が信じられないような誤解をして、全然理解できなく、目が回っているいるような印象を受けたこと、
相当ゼロ除算の研究をされている方が、基本を誤解されていたこと、1/0 の定義を誤解されていた。
相当な才能の持ち主が、連続性や順序に拘って、4年以上もゼロ除算の研究を避けていたこと。
これらのことは、人間如何に予断と偏見にハマった存在であるかを教えている。
まずは ゼロ除算は不可能であるの 思いが強すぎで、初めからダメ、考えない、無視の気持ちが、強い。 ゼロ除算を従来の 掛け算の逆と考えると、不可能であるが 証明されてしまうので、割り算の意味を拡張しないと、考えられない。それで、 1/0,0/0,z/0 などの意味を発見する必要がある。 それらの意味は、普通の意味ではないことの 初めの考えを飛ばして ダメ、ダメの感情が 突っ走ている。 非ユークリッド幾何学の出現や天動説が地動説に変わった世界史の事件のような 形相と言える。
2018.9.22.6:41
ゼロ除算の4つの誤解:
1. ゼロでは割れない、ゼロ除算は 不可能である との考え方に拘って、思考停止している。 普通、不可能であるは、考え方や意味を拡張して 可能にできないかと考えるのが 数学の伝統であるが、それができない。
2. 可能にする考え方が 紹介されても ゼロ除算の意味を誤解して、繰り返し間違えている。可能にする理論を 素直に理解しない、 強い従来の考えに縛られている。拘っている。
3. ゼロ除算を関数に適用すると 強力な不連続性を示すが、連続性のアリストテレス以来の 連続性の考えに囚われていて 強力な不連続性を受け入れられない。数学では、不連続性の概念を明確に持っているのに、不連続性の凄い現象に、ゼロ除算の場合には 理解できない。
4. 深刻な誤解は、ゼロ除算は本質的に定義であり、仮定に基づいているので 疑いの気持ちがぬぐえず、ダメ、怪しいと誤解している。数学が公理系に基づいた理論体系のように、ゼロ除算は 新しい仮定に基づいていること。 定義に基づいていることの認識が良く理解できず、誤解している。
George Gamow (1904-1968) Russian-born American nuclear physicist and cosmologist remarked that "it is well known to students of high school algebra" that division by zero is not valid; and Einstein admitted it as {\bf the biggest blunder of his life} [1]:1. Gamow, G., My World Line (Viking, New York). p 44, 1970.